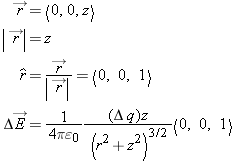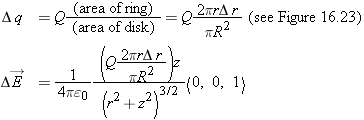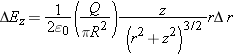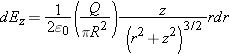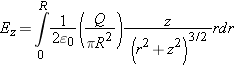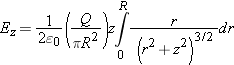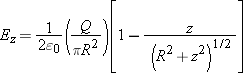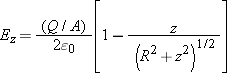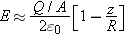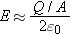|
A Uniformly Charged Disk |
 |
One reason that a uniformly charged disk is important is that two oppositely charged metal disks can form a “capacitor,” a
device that is important in electric circuits. Before discussing metal disks, we will consider a glass disk that has been
rubbed with silk in such a way as to deposit a uniform density of positive charge all over the surface.
Field Along the Axis of a Uniformly Charged Disk
We consider a disk of radius R, with a total charge Q uniformly distributed over the front surface of the disk.
Step 1: Cut Up the Charge Distribution into Pieces; Draw 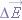
Use thin concentric rings as pieces, as shown in Figure
16.22, since we already know the electric field of a uniform ring. Approximate each ring as having some average radius
r.
Step 2: Write an Expression for the 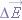 Due to One Piece
Due to One Piece
Origin: Center of ring.
Location of piece: Given by radius r of ring.
Note that both Δq and r will be different for each piece.
Components to calculate: Only Δ
Ez is nonzero.
or, for infinitesimally thin rings:
Step 3: Sum All Contributions
Many of these quantities have the same values for different values of
r, and these can be taken out of the integral as common factors:
This particular integral can be done by a change of variables, letting
u = (
r2 +
z2). You can work it out yourself, look up the result in a table of integrals, or use a symbolic math package or calculator
to evaluate it. The result is:
for a uniformly charged disk of charge
Q and radius
R, at locations along the axis of the disk. This is often written in terms of the area
A of the disk (
A =
π R2):
Step 4: Check
Direction: Away from the disk if Q is positive, as expected.
Special location: 0 <<
z <<
R (very close to the disk, but not touching it. See Figure
16.25.)
 |
 |
|
|
 |
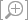
|
Figure 16.25 |
Magnitude of electric field along the axis of a disk, for z < 0.1R.
|
|
 |
|
 |
Although we proved this result only for observation locations a perpendicular distance
z <<
R from the center of the disk, the result is actually a good approximation as long as you're not too near the edge of the disk,
as can be seen in Figure
16.21, which is the result of an accurate numerical integration.
| 16.X.5 |
For a disk of radius R = 20 cm and Q = 6 × 10 −6 C, calculate the electric field 2 mm from the center of the disk using all three formulas:
How good are the approximate formulas at this distance? For the same disk, calculate E at a distance of 5 cm (50 mm) using all three formulas. How good are the approximate formulas at this distance?
|
|
 |
 |
 |
|
|
|
|
 |
| Copyright © 2011 John Wiley & Sons, Inc. All rights reserved. |
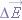
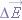 Due to One Piece
Due to One Piece