16.2.
Periodic Waves
Three quantities are needed to describe periodic waves: period, wavelength, and amplitude. The wavelength and amplitude can be found from a space representation of the wave which is essentially a "snapshot" taken of the wave at an instant in time.
The period and amplitude can be found from a time representation of the wave. This is constructed by making a graph of the motion of a point in the medium. The graph is the displacement of the point from its undisturbed position versus time. This is equivalent to making a vertical position versus time graph of a small cork bobbing up and down in water as a wave passes by.
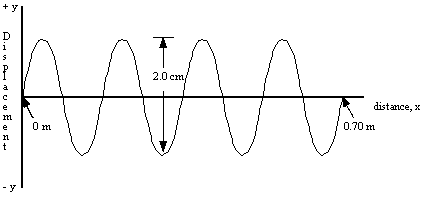
Example 1
A snapshot is taken of a periodic wave traveling on a string as shown below. What is the amplitude and wavelength of the wave?
The height of the wave is seen from the "snapshot" to be 2.0 cm. The amplitude is only one-half of this, so
| A = 1.0 cm. |  |
The wave is seen to have four wavelengths spread over a distance of 0.70 m so the wavelength
|
l = 0.70 m/4 = 0.18 m. | |
Example 2
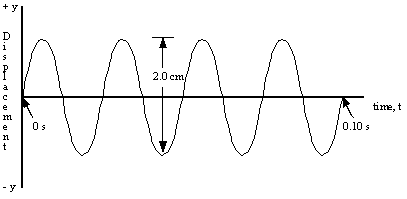
A point in the medium carrying the wave in example 1 is observed and a displacement versus time graph is made and shown below. Find the period, frequency, and amplitude of the wave from this graph. What is the speed of the wave?
Again, the amplitude is one-half of the total height of the sine wave,
| A = 1.0 cm. | |
The period of the wave is found by observing that four complete oscillations occur in 0.10 s, so
| T = (0.10 s)/4 = 0.025 s. | |
The frequency of the wave is then
| f = 1/T = 1/0.025 s = 4.0 × 101 Hz. | |
The speed of the wave is
| v =lf = (0.18 m)(4.0 × 101 Hz) = 7.2 m/s. | |
Example 3
Radio waves travel with a speed of 3.00 × 108 m/s. What is the wavelength of AM radio waves whose frequency is 640 kHz?
Equation (16.1) is true for waves of all kinds, including radio waves. The wavelength of the AM waves is
|
l = v/f = (3.00 × 108 m/s)/(640 × 103 Hz) = 470 m. | |
|
| Copyright © 2000-2003 by John Wiley & Sons, Inc. or related companies. All rights reserved. |
