|
In the x direction, the spacecraft in Figure 3.5 has an initial velocity component of v0x =+22 m/s and an acceleration component of ax=+24 m/s2. In the y direction, the analogous quantities are v0y=+14 m/s and ay=+12 m/s2. The directions to the right and upward have been chosen as the positive directions. Find (a) x and vx, (b) y and vy, and (c) the final velocity (magnitude and direction) of the spacecraft at time t=7.0 s.
Reasoning
The motion in the x direction and the motion in the y direction can be treated separately, each as a one-dimensional motion. We will follow this approach in parts (a) and (b) to obtain the location and velocity components of the spacecraft. Then, in part (c) the velocity components will be combined to give the final velocity.
Problem solving insight
When the motion is two-dimensional, the time variable t has the same value for both the x and y directions. |
|
Solution
| (a) |
The data for the motion in the x direction are listed below.
x-Direction Data
|
x
|
ax
|
vx
|
v0x
|
t
|
?
|
+24 m/s2
|
?
|
+22 m/s
|
7.0 s
|
The x component of the craft’s displacement can be found by using Equation 3.5a.
The velocity component vx can be calculated with the aid of Equation 3.3a:
| | (b) |
The data for the motion in the y direction are listed below.
y-Direction Data
|
y
|
ay
|
vy
|
v0y
|
t
|
?
|
+12 m/s2
|
?
|
+14 m/s
|
7.0 s
|
Proceeding in the same manner as in part (a), we find that
| | (c) |
Figure 3.7 shows the velocity of the vehicle and its components vx and vy. The magnitude v of the velocity can be found by using the Pythagorean theorem:
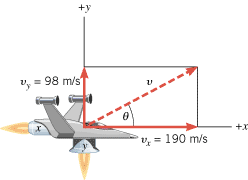 | | Figure 3.7
The magnitude of the velocity vector gives the speed of the spacecraft, and the angle q gives the direction of travel relative to the positive x direction. |
|
The direction of the velocity vector is given by the angle q in the drawing:
After 7.0 s, the spacecraft has a velocity of 210 m/s in a direction of 27° above the positive x axis. The craft is 740 m to the right and 390 m above the origin, as in Figure 3.5.
|
|
