Example 1 A Tire Pressure Gauge

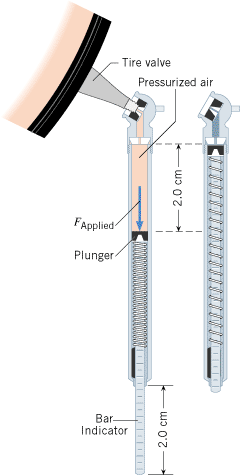
In a tire pressure gauge, the air in the tire pushes against a plunger attached to a spring when the gauge is pressed against the tire valve, as in Figure 10.2. Suppose the spring constant of the spring is k=320 N/m and the bar indicator of the gauge extends 2.0 cm when the gauge is pressed against the tire valve. What force does the air in the tire apply to the spring?

Figure 10.2 In a tire pressure gauge, the pressurized air from the tire exerts a force F _Applied that compresses a spring.

Reasoning We assume that the spring is an ideal spring, so that the relation is obeyed. The spring constant k is known, as is the displacement x. Therefore, we can determine the force applied to the spring.

Solution The force needed to compress the spring is given by Equation 10.1 as

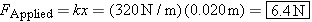Thus, the exposed length of the bar indicator indicates the force that the air pressure in the tire exerts on the spring. We will see later that pressure is force per unit area, so force is pressure times area. Since the area of the plunger surface is fixed, the bar indicator can be marked in units of pressure.