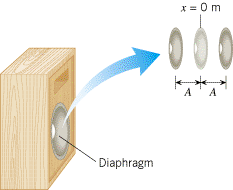
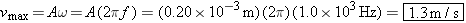The diaphragm of a loudspeaker moves back and forth in simple harmonic motion to create sound, as in Figure 10.12. The frequency of the motion is f=1.0 kHz and the amplitude is A=0.20 mm. (a) What is the maximum speed of the diaphragm? (b) Where in the motion does this maximum speed occur?

Figure 10.12  The diaphragm of a loudspeaker generates a sound by moving back and forth in simple harmonic motion.

Reasoning  The maximum speed v_max of an object vibrating in simple harmonic motion is v_max=Aw (w in rad/s), according to Equation 10.8. The angular frequency w is related to the frequency f by w=2pf, according to Equation 10.6.

Solution

(a)	Using Equations 10.8 and 10.6, we find that the maximum speed of the vibrating diaphragm is
(b)	The speed of the diaphragm is zero when the diaphragm momentarily comes to rest at either end of its motion: x=+A and x=–A. Its maximum speed occurs midway between these two positions, or at x=0 m.