Suppose the person singing in the shower in Figure 16.25 produces a sound power P. Sound reflects from the surrounding shower stall. At a distance r in front of the person, does Equation 16.9, I=P/(4pr²), underestimate, overestimate, or give the correct sound intensity?

Figure 16.25  When someone sings in the shower, the sound power passing through part of an imaginary spherical surface (shown in blue) is the sum of the direct sound power and the reflected sound power.

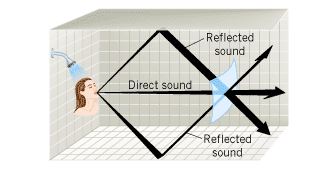
Reasoning and Solution In arriving at Equation 16.9, it was assumed that the sound spreads out uniformly from the source and passes only once through the imaginary surface that surrounds it (see Figure 16.23). Only part of this imaginary surface is shown in Figure 16.25. The drawing illustrates three paths by which the sound passes through the surface. The “direct” sound travels directly along a path from its source to the surface. It is the intensity of the direct sound that is given by I=P/(4pr²). The remaining paths are two of the many that characterize the sound reflected from the shower stall. The total sound power that passes through the surface is the sum of the direct and reflected powers. Thus, the total sound intensity at a distance r from the source is greater than that of the direct sound alone, and the relation I=P/(4pr²) underestimates the sound intensity from the singing because it does not take into account the reflected sound. People like to sing in the shower because their voices sound so much louder due to the enhanced intensity caused by the reflected sound.

Related Homework: Problems 52, 64