2.7 Kepler's Laws of Planetary Motion
Pre-Lecture Reading 2.7
-
•Astronomy Today, 8th Edition (Chaisson & McMillan)
-
•Astronomy Today, 7th Edition (Chaisson & McMillan)
-
•Astronomy Today, 6th Edition (Chaisson & McMillan)
Video Lecture
-
•Kepler's Laws of Planetary Motion (26:56)
Supplementary Notes
Kepler's First Law (KI)
-
•See Kepler's First Law (KI).
The orbital paths of the planets are elliptical (not circular), with the sun at one focus.
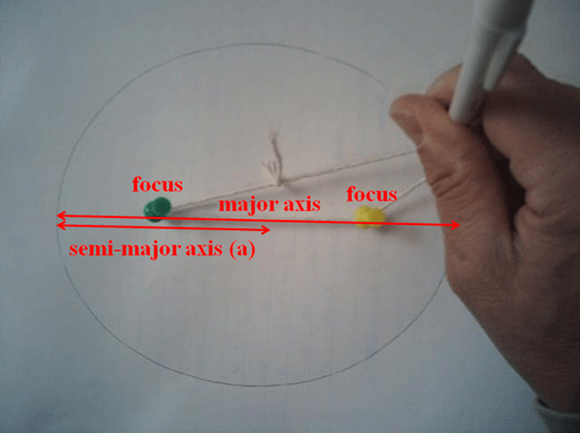
Figure 1: Ellipse terminology
-
•KI destroyed the Platonic/Aristotelian aesthetic of circular motion.
Kepler's Second Law (KII)
An imaginary line connecting the sun to any planet sweeps out equal areas of the ellipse in equal intervals of time.
-
•This is an obscure, yet compact, geometrical way of stating that planets orbit faster when close to the sun and slower when farther away, and specifically how much faster and slower.
-
•KII destroyed the Platonic/Aristotelian aesthetic of uniform motion.
Kepler's Third Law (KIII)
The square of a planet's orbital period, P, is proportional to the cube of its semi-major axis, a.
-
•KII tells us when a planet is moving faster or slower, but does not tell us how long it takes to complete an orbit. KIII does this, as a function of the orbit's semi-major axis.
-
•Mathematically, KIII is written:
P2 α a3
-
•This means:
P2 = constant × a3
-
•Kepler could measure this constant of proportionality, but could not explain why it has the value that it does. That would have to wait for Newton (see Derivation of Kepler's Laws from Newton's Law).
-
•KIII also holds for objects in orbit around objects other than the sun, such as the moons of Jupiter in orbit around Jupiter, Earth's moon in orbit around Earth, etc., but with a different constant of proportionality for each central body (e.g., constantsun, constantJupiter, constantEarth, etc.).
Applications of Kepler's Third Law
Many equations in this course, including KIII, are significantly easier to use if they are rewritten such that their inputs and outputs can be stated in terms of ratios with respect to a comparison object (instead of in terms of meters, seconds, etc.). We will consider two cases.Case 1. For objects in orbit around the sun, we will use Earth as a comparison object
-
•Consider the case of Earth orbiting the sun. For Earth, P = 1 yr and a = 1 AU. Hence, for Earth:
(1 yr)2 = constantsun × (1 AU)3
-
•Dividing this equation into the previous equation yields:
-
•Simplification yields:
-
•Notice that the constant of proportionality cancels out.
-
•Hence, as long as P is measured in years, a is measured in AU, and the object orbits the sun, KIII should be easy to use.
Example:
A planet orbits the sun at half of Earth's distance from the sun. Hence,
A planet orbits the sun at half of Earth's distance from the sun. Hence,
a = 0.5 AU
and P = 0.53/2 yr,
or 0.35 times as long as Earth takes to orbit the sun.
Case 2. For objects in orbit around Earth, we will use the moon as a comparison object
-
•Consider the case of the moon orbiting Earth. For the moon, P = 1 lunar month anda = 1Earth-moon distance. Hence, for the moon:
(1 lunar month)2 = constantEarth × (1 Earth-moon distance)3
-
•Dividing this equation into the previous equation yields:
-
•Simplification yields:
-
•Again, notice that the constant of proportionality cancels out.
-
•Hence, as long as P is measured in lunar months, a is measured in Earth-moon distances, and the object orbits Earth, KIII should be easy to use.
Example:
A satellite takes twice as long to orbit Earth as the moon takes to orbit Earth. Hence,
A satellite takes twice as long to orbit Earth as the moon takes to orbit Earth. Hence,
P = 2
lunar months and a = 22/3 Earth-moon distances, or 1.6 times the semi-major axis of the moon's orbit.
Exercises
-
•Experiment with UNL's Eccentricity Demonstrator.
-
•Experiment with UNL's Planetary Orbits Simulator.
-
•Experiment with UNL's Kepler's Third Law.
Assignment 2
-
•Do Questions 5 and 6.