Appendix A – Reporting Quantitative Measurements and Results

Introduction

A numerical measurement indicates the number of units in a measurement. For example, a measurement in time could indicate the number of seconds, minutes, years, etc. Thus, units must always be reported with a measurement. The number of digits used to report the number of units indicates how precisely the measurement was made. Thus, care must be taken to report the result to the correct number of significant figures. In this appendix, we discuss how quantitative measurements should be reported.

A.1 Precision

Introduction

The precision of a measurement is indicated by the number of significant figures in the reported number.

Objectives

•
Report the result of a measurement to the correct number of digits.

A.1-1. Precision

The last digit in a measurement should always be an estimate.

The precision of a measurement is given by the number of digits to which the numerical value is reported. It is normally dictated by the measuring device. The last digit of a reported measurement is usually an estimate, and, unless stated otherwise, it is generally assumed good to 1 unit. Thus, if a mass is reported to be 3 g, the reader will assume that the mass is somewhere between 2 and 4 g. A reported mass of 3.0 g tells the reader that the measurement was made more precisely, and that the mass is between 2.9 and 3.1 g. Thus, 3 and 3.0 may represent the same magnitude, but they indicate a difference in the precision of the measurement.

A.1-2. Example

Exercise A.1:

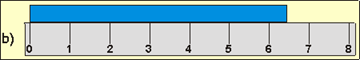
Indicate the length of the blue line to the correct precision for each measuring device.

6_1__ The blue bar is a little over 3/4 the length of the measuring device. The measuring device is 8 units long, so the blue bar is 6 or 7 units in length. units

6.4_0.10000001__ The blue bar is slightly less than half way between 6 and 7 units, so it 6.4 or 6.5 units long. units

6.43_0.010000001__ The end of the blue bar is about 1/3 of the way between 6.4 and 6.5, so it is 6.43 units long. units

A.2 Significant Figures

Introduction

Not all digits in a number are necessarily significant. In this section, we see how to determine those that are.

Objectives

•
Determine the number of significant figures in a number.

A.2-1. Introduction

Significant figures are the digits that are obtained in a measurement. Thus, the precision of a measurement is indicated by the number of significant figures it contains. A measurement of 6.43 cm, which contains three significant figures, is more precise than a measurement of 6.4 cm, which contains only two significant figures. Reporting the number of significant figures in a measurement correctly is important because the number of significant figures indicates the precision of the measurement. The most common mistake made in reporting a measurement is not reporting trailing zeros to the right of the decimal. However, in a science laboratory, there is a big difference between a measurement reported to be 3 g and one reported as 3.0000 g. It is important that your number show both the magnitude and precision correctly. Consider the case where you are trying to prove or disprove a prediction that the mass of the product of a reaction should be 2.80 g. A measurement of 3.0000 g disproves the prediction, but a measurement of 3 g is inconclusive.

A.2-2. Rules

There are some simple rules that allow us to determine which digits in a number are significant.

1
All nonzero numbers in a reported measurement are significant.
2
Zeroes to the left of the decimal but to the right of all nonzero digits cannot be assumed significant. In this course, we use the practice of placing a decimal at the end of a number to indicate that the zeroes are significant. Thus, the number of significant figures in the number '300' is unclear while the number '300.' has three significant figures. The best way to indicate the number of significant figures is to use scientific notation. The numbers 3e+02 (3 × 10²), 3.0e+02 (3.0 × 10²), and 3.00e+02 (3.00 × 10²) show a measurement of 300 to one, two and three significant figures, respectively.
3
Leading zeroes for numbers less than one are not significant, but other zeros in the number are significant. The number 0.00012 contains only two significant figures. This becomes apparent when the number is expressed in scientific notation, 1.2e–04 (1.2 × 10^–4).
4
All zeroes to the right of the decimal of numbers greater than one are significant. The number 1.00012 contains six significant figures. If you are uncertain about the number of significant figures in a number, rewrite the number in scientific notation. All digits of a number expressed in scientific notation are significant.

A.2-3. Example

Exercise: A.2

Number	Significant Digits
3.000	4_0__ Rule 4 indicates that all zeroes to the right of the decimal are significant.
320	2_0__ Rule 2 indicates that zeroes to the left of the decimal but to the right of all nonzero digits cannot be assumed to be significant.
0.0005606	4_0__ Rule 3 indicates that leading zeroes for numbers less than 1 are not significant.
400.	3_0__ We use a decimal at the end of a number that ends with zero to indicate that all numbers to the left of the decimal are significant.

A.3 Reporting Answers to Calculations

Introduction

It is frequently the case that the number to be reported is not the measurement itself, but a number obtained after a calculation involving several measurements. As with individual measurements, it is important to report the result of a calculation to the correct number of significant figures so that the reader understands the precision to which the result is known.

Objectives

•
Report the result of a calculation to the correct number of significant figures.

A.3-1. Introduction

A common mistake in reporting results of a calculation is to include all of the digits shown on the calculator. For example, consider a 5.2 mL sample that has a mass of 3.7 g. The density of the material would be determined to be

d =

3.7 g

5.2 mL

The result of 3.7/5.2 on many calculators is 0.711538, but if you report the density with that many significant figures, you would imply far more precision in your measurements than is warranted by the experiment. Thus, the answer must be rounded to the correct number of significant figures. The following two rules should help you report the result of a calculation correctly.

1
Multiplications and Divisions: The number of significant figures in the result of a calculation involving multiplication or division is equal to the number of significant figures in the least precise number used in the calculation. Thus, the density discussed in the preceding paragraph should be rounded to 0.71 g/mL because both the mass and the volume were measured to two significant figures.
2
Additions and Subtractions: The number of decimal places in the result of an addition or subtraction is equal to the number of decimal places in the given number that has the fewest decimal places. A good way to remember this rule is to realize that the result of the addition of a significant number and an insignificant number is insignificant. If you had $3.25 and someone gave you about $2 in change, you would have a total of about $5, not $5.25, but if they gave you $2.00 in change, you would have $5.25.

A.3-2. Example

Exercise: A.3

Express each result to the correct number of significant figures.

Operation

Calculator

Result

(2.7)(6.345)

17.1315

17.1315__2_ The number of significant figures in the result of a multiplication equals the number of significant figures in the number with the least number of significant figures. 2.7 has only two significant figures.

1.0 – 0.0003

0.9997

0.9997___1 1.0 is significant only to a tenth, so the answer is significant to only a tenth.

12.3 – 11.2634

1.0366

1.0366_.0366__1 12.3 is significant to only a tenth, so the answer is significant to only a tenth.

8.76 + 7.13

15.89

15.89___2 Both numbers are significant to the hundredths place, so the answer is significant to the hundredths place.

8.5128/3.20

2.66025

2.66025__3_ 3.20 has only three significant figures, so the answer can have only three significant figures.

(12.3425 – 12.3417)

23.2268

3.444297e–05

3.444297e-05_5e-6_1_ The result of the subtraction is 0.0008, which has only one significant figure. Thus, the result of the division can have only one significant figure.

A.4 Rounding Errors

Introduction

When intermediate values in a calculation involving several steps must also be reported, they should be reported to the correct number of significant figures. However, use of rounded values in subsequent calculations can lead to significant rounding errors.

Objectives

•
Minimize rounding errors in consecutive calculations.

A.4-1. Example

Do not use the rounded values for intermediate results when doing sequential calculations.

Consider the following example of rounding error.

Example:
A mixture contains 4.0 g of N₂ (M_m = 28.0 g/mol) and 4.0 g of O₂ (M_m = 32.0 g/mol).

How many moles of each gas are present in the mixture? Divide the mass by the molar mass to obtain the number of moles of each gas. The results of the calculation as shown on a calculator are: moles of N₂ = 4.0/28.0 = 0.14286 mol and moles of O₂ = 4.0/32.0 = 0.125 mol. However each answer is good to only two significant figures, so the number of moles of each gas would be rounded to 0.14 mol N₂ and 0.13 mol O₂. What is value of the ratio of moles of O₂ to moles of N₂ in the mixture?

Using the rounded intermediate values:

0.13 mol O₂

0.14 mol N₂

= 0.93 mol O₂/mol N₂

With no rounding of intermediate values:

4.0/32.0 mol O₂

7.0/28.0 mol N₂

= 0.88 mol O₂/ mol N₂

The two answers differ by 6% as a result of rounding errors. As demonstrated in the example, rounding errors can be substantial, and it can get worse in calculations involving several steps. Consequently, calculations with rounded numbers should be avoided whenever possible. If rounded numbers must be used, they should be used with more digits than can be expected for the final answer. We show many intermediate answers in this course, which have been rounded to the correct number of significant figures, but the final answer that is given is always calculated without the use of the rounded numbers. You should always keep that in mind when you compare your answers with those given or expected.